Power Law distributions

A power law distribution is a type of probability distribution that follows a pattern where a small number of items (such as events or entities) have a disproportionately large number of occurrences, while many items have very few occurrences. This kind of distribution is also known as a “scale-free“ distribution because it does not have a characteristic scale, and it is often found in Complex systems exhibit emergent behavior such as the internet, social networks, and the distribution of wealth.

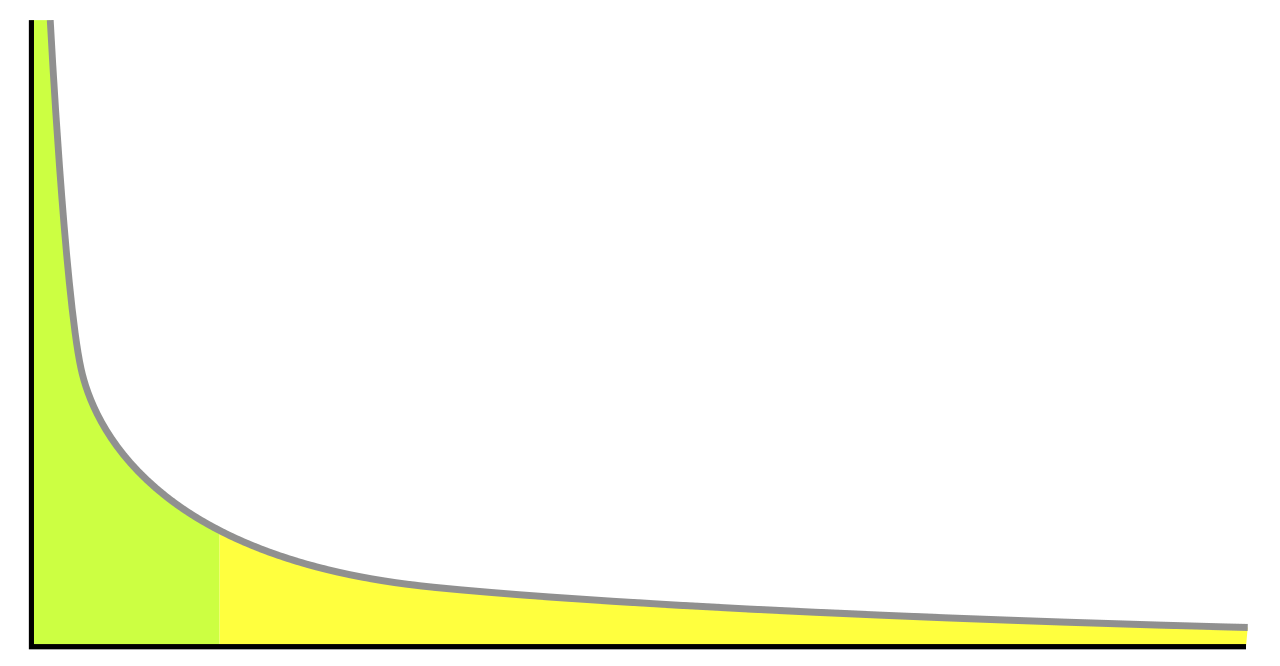
An example of a power-law graph showing the long tail 80-20 distribution (Pareto Principle).

from Linked – Barabási, Frangos (2002):

a histogram following a power law is a continuously decreasing curve, implying that many small events coexist with a few large events.
…
If the heights of an imaginary planet’s inhabitants followed a power law distribution, most creatures would be really short. But nobody would be surprised to see occasionally a hundred-feet-tall monster walking down the street. In fact, among six billion inhabitants there would be at least one over 8,000 feet tall.
…
Power laws formulate in mathematical terms the notion that a few large events carry most of the action.
…
But power laws are at the heart of some of the most stunning conceptual advances in the second half of the twentieth century, emerging in fields like chaos, fractals, and phase transitions. Spotting them in networks signaled unsuspected links to other natural phenomena and placed networks at the forefront of our understanding of Complex systems exhibit emergent behavior in general.

#complexity #systems #networks #mathematics